The Principle of Indifference (Insufficient Reason)
There are several wordings of The Principle of Insufficient Reason
(Indifference), all saying much the same thing. The authors are -with good reason- usually being critical
of it.
Keynes (1920) states it as
If there is no known reason for predicating
of our subject one rather than another of several alternatives, then
relative to such knowledge the assertations of each of these
alternatives have an equal probability. Thus equal
probabilities must be assigned to each of several arguments, if there is
an absence of positive ground for assigning unequal ones.
Hájek (2012) gives it as
whenever there is no evidence favoring one
possibility over another, they have the same probability
Wikipedia
puts it slightly differently:-
Suppose that there are n>1 mutually exclusive and
collectively exhaustive possibilities. The principle of indifference
states that if the n possibilities are indistinguishable except for
their names, then each possibility should be assigned a probability
equal to 1/n.
The principle, as worded above in all of the quoted versions, is claimed to
give actual probabilities, but no proof of that claim is given.
There is a theorem (see the next box), but that theorem is about what probabilities do on average (that is, about likelinesses), not
about individual probabilities, themselves.
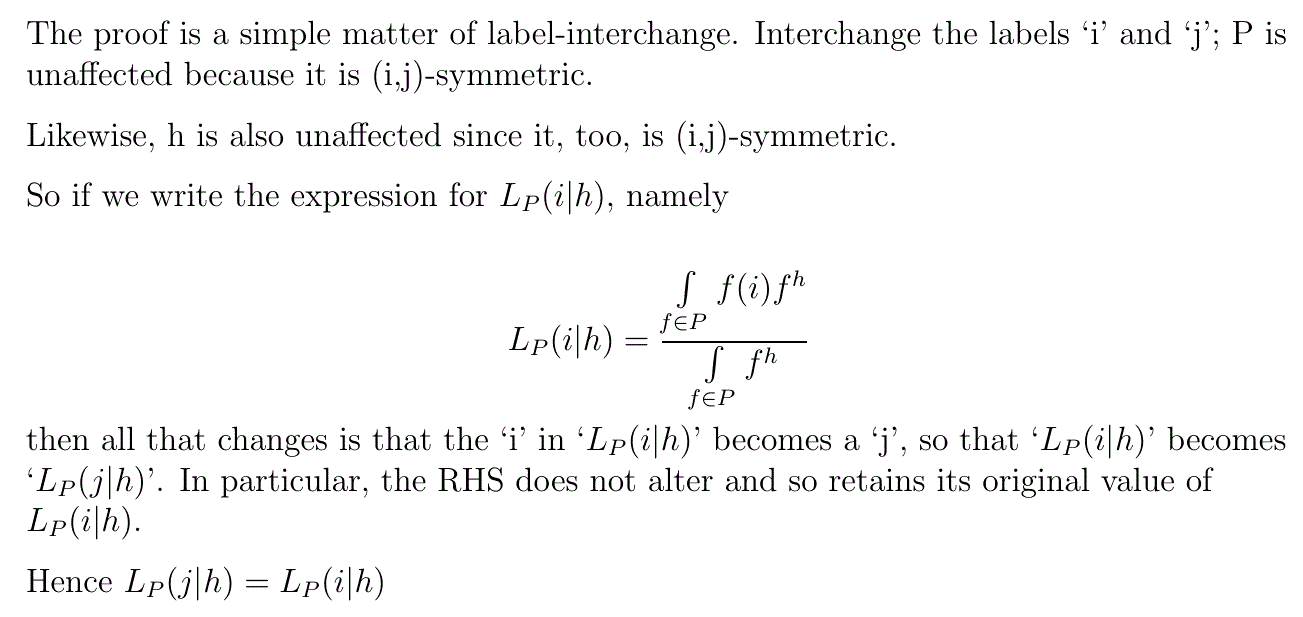
The Indifference Theorem
(Theorem of Insufficient Reason)
Let P be an underlying set in S(N), i,j∈X
N and h∈H(N).
If P and h are both (i,j)-symmetric then L
P(j|h)=L
P(i|h).
In particular, let c be any constant histogram
and P be symmetric. Then LP(i|c)=1/N
for all i since LP(i|c)=LP(1|c)
by (i,1)-symmetry. For example,
LS(N)(i)=1/N for all i.